Center of curvature

A concave mirror with light rays
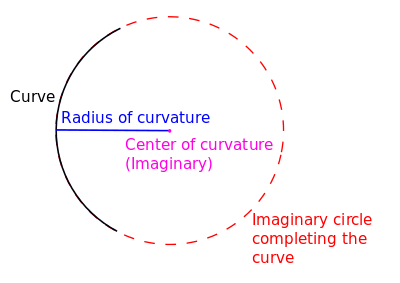
Center of curvature
In geometry, the center of curvature of a curve is found at a point that is at a distance from the curve equal to the radius of curvature lying on the normal vector. It is the point at infinity if the curvature is zero. The osculating circle to the curve is centered at the centre of curvature. Cauchy defined the center of curvature C as the intersection point of two infinitely close normal lines to the curve.[1] The locus of centers of curvature for each point on the curve comprise the evolute of the curve.
See also
Ref-notes
- ↑
- Borovik, Alexandre; Katz, Mikhail G. (2011), "Who gave you the Cauchy--Weierstrass tale? The dual history of rigorous calculus", Foundations of Science, arXiv:1108.2885
 , doi:10.1007/s10699-011-9235-x
, doi:10.1007/s10699-011-9235-x
- Borovik, Alexandre; Katz, Mikhail G. (2011), "Who gave you the Cauchy--Weierstrass tale? The dual history of rigorous calculus", Foundations of Science, arXiv:1108.2885
References
- Hilbert, David; Cohn-Vossen, Stephan (1952), Geometry and the Imagination (2nd ed.), New York: Chelsea, ISBN 978-0-8284-0087-9
This article is issued from Wikipedia - version of the 1/23/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.