Pompeiu derivative

In mathematical analysis, a Pompeiu derivative is a real-valued function of one real variable that is the derivative of an everywhere differentiable function and that vanishes in a dense set. In particular, a Pompeiu derivative is discontinuous at any point where it is not 0. Whether non-identically zero such functions may exist was a problem that arose in the context of early-1900s research on functional differentiability and integrability. The question was affirmatively answered by Dimitrie Pompeiu by constructing an explicit example; these functions are therefore named after him.

Pompeiu's construction

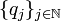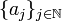Pompeiu's construction is described here. Let $\sqrt[3]{x}$ denote the real cubic root of the real number $x.$ Let $\{q_j\}_{j\in \N}$ be an enumeration of the rational numbers in the unit interval $[0,\,1].$ Let $\{a_j\}_{j\in \N}$ be positive real numbers with 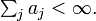$\textstyle\sum_j a_j < \infty.$ Define, for all $x\in [0,\,1]$

$g(x):=\sum_{j=0}^\infty \,a_j \sqrt[3]{x-q_j}.$

Since for any $x\in[0,\,1]$ each term of the series is less than or equal to a_j in absolute value, the series uniformly converges to a continuous, strictly increasing function g(x), due to the Weierstrass M-test. Moreover, it turns out that the function g is differentiable, with

$g^{\prime}(x):=\frac{1}{3}\sum_{j=0}^\infty \frac{a_j}{\sqrt[3]{(x-q_j)^2}}>0,$

at any point where the sum is finite; also, at all other points, in particular, at any of the 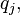$q_j,$ one has 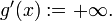$\textstyle g^{\prime}(x):=+\infty.$ Since the image of 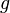$g$ is a closed bounded interval with left endpoint 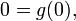$0=g(0),$ up to a multiplicative constant factor one can assume that g maps the interval $[0,\,1]$ onto itself. 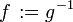Since g is strictly increasing, it is a homeomorphism; and by the theorem of differentiation of the inverse function, its composition inverse $f\,:=g^{-1}$ has a finite derivative at any point, which vanishes at least in the points 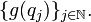$\{g(q_j)\}_{j\in \N}.$ These form a dense subset of $[0,\,1]$ (actually, it vanishes in many other points; see below).

Properties

• It is known that the zero-set of a derivative of any everywhere differentiable function is a G_δ subset of the real line. By definition, for any Pompeiu function this set is a dense G_δ set, therefore by the Baire category theorem it is a residual set. In particular, it possesses uncountably many points.
• A linear combination af(x) + bg(x) of Pompeiu functions is a derivative, and vanishes on the set {f = 0} ∩ {g = 0}, which is a dense G_δ by the Baire category theorem. Thus, Pompeiu functions are a vector space of functions.
• A limit function of a uniformly convergent sequence of Pompeiu derivatives is a Pompeiou derivative. Indeed, it is a derivative, due to the theorem of limit under the sign of derivative. Moreover, it vanishes in the intersection of the zero sets of the functions of the sequences: since these are dense G_δ sets, the zero set of the limit function is also dense.
• As a consequence, the class E of all bounded Pompeiu derivatives on an interval [a, b] is a closed linear subspace of the Banach space of all bounded functions under the uniform distance (hence, it is a Banach space).
• Pompeiu's above construction of a positive function is a rather peculiar example of a Pompeiu's function: a theorem of Weil states that generically a Pompeiu derivative assumes both positive and negative values in dense sets, in the precise meaning that such functions constitute a residual set of the Banach space E.

References

• Pompeiu, Dimitrie, "Sur les fonctions dérivées"; Math. Ann. 63 (1907), no. 3, 326—332.
• Andrew M. Bruckner, "Differentiation of real functions"; CRM Monograph series, Montreal (1994).