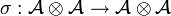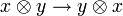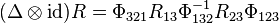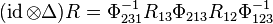Quasi-triangular quasi-Hopf algebra
A quasi-triangular quasi-Hopf algebra is a specialized form of a quasi-Hopf algebra defined by the Ukrainian mathematician Vladimir Drinfeld in 1989. It is also a generalized form of a quasi-triangular Hopf algebra.
A quasi-triangular quasi-Hopf algebra is a set 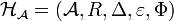 where
where 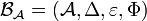 is a quasi-Hopf algebra and
is a quasi-Hopf algebra and 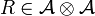 known as the R-matrix, is an invertible element such that
known as the R-matrix, is an invertible element such that
so that 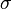 is the switch map and
is the switch map and
where 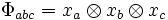 and
and 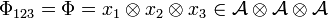 .
.
The quasi-Hopf algebra becomes triangular if in addition, 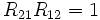 .
.
The twisting of  by
by 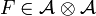 is the same as for a quasi-Hopf algebra, with the additional definition of the twisted R-matrix
is the same as for a quasi-Hopf algebra, with the additional definition of the twisted R-matrix
A quasi-triangular (resp. triangular) quasi-Hopf algebra with 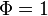 is a quasi-triangular (resp. triangular) Hopf algebra as the latter two conditions in the definition reduce the conditions of quasi-triangularity of a Hopf algebra.
is a quasi-triangular (resp. triangular) Hopf algebra as the latter two conditions in the definition reduce the conditions of quasi-triangularity of a Hopf algebra.
Similarly to the twisting properties of the quasi-Hopf algebra, the property of being quasi-triangular or triangular quasi-Hopf algebra is preserved by twisting.
See also
References
- Vladimir Drinfeld, "Quasi-Hopf algebras", Leningrad mathematical journal (1989), 1419–1457
- J. M. Maillet and J. Sanchez de Santos, "Drinfeld Twists and Algebraic Bethe Ansatz", American Mathematical Society Translations: Series 2 Vol. 201, 2000