Triangular function

Triangular function

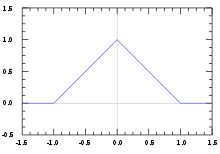
The triangular function (also known as the triangle function, hat function, or tent function) is defined either as:

$\begin{align} \operatorname{tri}(t) = \and (t) \quad &\overset{\underset{\mathrm{def}}{}}{=} \ \max(1 - |t|, 0) \\ &= \begin{cases} 1 - |t|, & |t| < 1 \\ 0, & \mbox{otherwise} \end{cases} \end{align}$

or, equivalently, as the convolution of two identical unit rectangular functions:

$\begin{align} \operatorname{tri}(t) = \operatorname{rect}(t) * \operatorname{rect}(t) \quad &\overset{\underset{\mathrm{def}}{}}{=} \int_{-\infty}^\infty \mathrm{rect}(\tau) \cdot \mathrm{rect}(t-\tau)\ d\tau\\ &= \int_{-\infty}^\infty \mathrm{rect}(\tau) \cdot \mathrm{rect}(\tau-t)\ d\tau . \end{align}$

The triangular function can also be represented as the product of the rectangular and absolute value functions:

$\operatorname{tri}(t) = \operatorname{rect}(t/2) \left ( 1 - \left |t \right | \right )$

The function is useful in signal processing and communication systems engineering as a representation of an idealized signal, and as a prototype or kernel from which more realistic signals can be derived. It also has applications in pulse code modulation as a pulse shape for transmitting digital signals and as a matched filter for receiving the signals. It is also equivalent to the triangular window sometimes called the Bartlett window.

Scaling

For any parameter, $a \ne 0\,$ :

$\begin{align} \operatorname{tri}(t/a) &= \int_{-\infty}^\infty \mathrm{rect}(\tau) \cdot \mathrm{rect}(\tau - t/a)\ d\tau \\ &= \begin{cases} 1 - |t/a|, & |t| < |a| \\ 0, & \mbox{otherwise} . \end{cases} \end{align}$

Fourier transform

The transform is easily determined using the convolution property of Fourier transforms and the Fourier transform of the rectangular function:

$\begin{align} \mathcal{F}\{\operatorname{tri}(t)\} &= \mathcal{F}\{\operatorname{rect}(t) * \operatorname{rect}(t)\}\\ &= \mathcal{F}\{\operatorname{rect}(t)\}\cdot \mathcal{F}\{\operatorname{rect}(t)\}\\ &= \mathcal{F}\{\operatorname{rect}(t)\}^2\\ &= \mathrm{sinc}^2(f), \end{align}$

where $\operatorname{sinc}(x) = \sin(\pi x) / (\pi x)$ is the normalized sinc function.

Extended version to repeat tent in all R domain

|(x mod 2)-1|

Alternative definition

Triangle function (alternate)

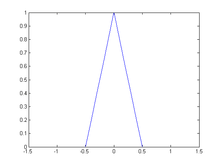
Note that in some cases the triangle function may be defined to have a base of length 1 instead of length 2:

$\begin{align} \operatorname{tri}(t) = \and (t) \quad &\overset{\underset{\mathrm{def}}{}}{=} \ \max(1 - |2t|, 0) \\ &= \begin{cases} 1 - |2t|, & |2t| < 1 \\ 0, & \mbox{otherwise} \end{cases} \end{align}$

See also

• Tent map
• Triangular distribution
• Triangle wave, a piecewise linear periodic function