Hexagonal crystal family
| Crystal system | Trigonal | Hexagonal | |
|---|---|---|---|
| Lattice system | 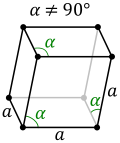 Rhombohedral |
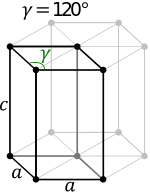 Hexagonal | |
| Example |  Dolomite |
 Quartz |
 Beryl |
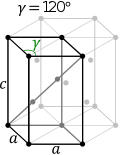
In crystallography, the hexagonal crystal family is one of the 6 crystal families. In the hexagonal family, the crystal is conventionally described by a right rhombic prism unit cell with two equal axes (a by a), an included angle of 120° (γ) and a height (c, which can be different from a) perpendicular to the two base axes.
The hexagonal crystal family consists of the 12 point groups such that at least one of their space groups has the hexagonal lattice as underlying lattice, and is the union of the hexagonal crystal system and the trigonal crystal system.[1] There are 52 space groups associated with it, which are exactly those whose Bravais lattice is either hexagonal or rhombohedral.
Lattice systems
The hexagonal crystal family consists of two lattice systems: hexagonal and rhombohedral. Each lattice system consists of one Bravais lattice.
| Bravais lattice | Hexagonal | Rhombohedral |
|---|---|---|
| Pearson symbol | hP | hR |
| Conventional hexagonal cell |  |
 |
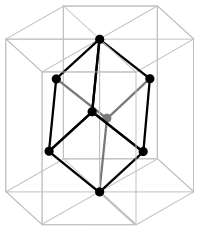
| Rhombohedral cell |  |
 |
The conventional cell for the rhombohedral Bravais lattice is the rhombohedrally-centered (R-centered) hexagonal cell, consisting of two additional lattice points which occupy the longest body diagonal of the unit cell with coordinates ( 2⁄3, 1⁄3, 1⁄3) and ( 1⁄3, 2⁄3, 2⁄3). Hence, there are 3 lattice points per unit cell in total and the lattice is non-primitive.
The Bravais lattices in the hexagonal crystal family can also be described by rhombohedral axes. The unit cell is a rhombohedron (which gives the name for the rhombohedral lattice system). This is a unit cell with parameters a = b = c; α = β = γ ≠ 90°.[2] In practice, the hexagonal description is more commonly used because it is easier to deal with a coordinate system with two 90° angles. However, the rhombohedral axes are often shown (for the rhombohedral lattice system) in textbooks because this cell reveals 3m symmetry of crystal lattice.
The unit cell for the hexagonal Bravais lattice in rhombohedral axes, consisting of two additional lattice points which occupy the longest body diagonal of the unit cell with coordinates ( 1⁄3, 1⁄3, 1⁄3) and ( 2⁄3, 2⁄3, 2⁄3), is called the hexagonally-centered (D-centered)[3] rhombohedral cell. However, such a description is rarely used.
Crystal systems
The hexagonal crystal family consists of two crystal systems: trigonal and hexagonal. A crystal system is a set of point groups in which the point groups themselves and their corresponding space groups are associated with a lattice system.
The trigonal crystal system consists of the 5 point groups that have a single three-fold rotation axis (see table in Crystal system#Crystal classes). Crystals in the rhombohedral lattice system are always in the trigonal crystal system, but some crystals such as alpha-quartz are in the trigonal crystal system but not in the rhombohedral lattice system (alpha-quartz is in the hexagonal lattice system). There are 25 space groups (143 to 167) whose corresponding point groups are one of the five in the trigonal crystal system, consisting of the seven space groups associated with the rhombohedral lattice system together with 18 associated with the hexagonal lattice system. The crystal structures of alpha-quartz in the previous example are described by two of those 18 space groups (152 and 154) associated with the hexagonal lattice system. (In total there are 45 space groups associated with the hexagonal lattice system, the remaining 27 space groups being part of the hexagonal crystal system to which another modification of quartz (β-quartz) belongs.)
The hexagonal crystal system consists of the seven point groups such that all their space groups have the hexagonal lattice as underlying lattice. Graphite is an example of a crystal that crystallizes in the hexagonal crystal system.
Comparison
| Crystal system | Required symmetries of point group | Point groups | Space groups | Lattice system |
|---|---|---|---|---|
| Trigonal | 1 threefold axis of rotation | 5 | 7 | Rhombohedral |
| 18 | Hexagonal | |||
| Hexagonal | 1 sixfold axis of rotation | 7 | 27 |
Rhombohedral lattice system vs Trigonal crystal system
The rhombohedral lattice system consists of the rhombohedral lattice, while the trigonal crystal system consists of the five point groups that have seven corresponding space groups associated with the rhombohedral lattice system (and 18 corresponding space groups associated with the hexagonal lattice system). An additional source of confusion is that all members of the trigonal crystal system with assigned rhombohedral lattice system (space groups 146, 148, 155, 160, 161, 166, and 167), can be represented with an equivalent hexagonal lattice with so called R-centering (rhombohedral-centering); there is a choice of using a R-centered hexagonal or a primitive rhombohedral setting for the lattice.[4][5]
Hexagonal lattice system vs Hexagonal crystal system
The hexagonal lattice system is associated with 45 space groups whose underlying lattice has point group of order 24, while the hexagonal crystal system consists of the 27 space groups such that all space groups with the same point group are in the hexagonal lattice system.
Crystal classes
Trigonal crystal system
The trigonal crystal system is the only crystal system whose point groups have more than one lattice system associated with their space groups: the hexagonal and rhombohedral lattices both appear.
The 5 point groups in this crystal system are listed below, with their international number and notation, their space groups in name and example crystals. (All these point groups are also associated with some space groups not in the rhombohedral lattice system.)[6][7][8]
| Space group no. | Point group | Examples | Space group | ||||
|---|---|---|---|---|---|---|---|
| Class | Intl | Schoen. | Orb. | Cox. | |||
| 143–146 | Pyramidal rhombohedral tetartohedral |
3 | C3 | 33 | [3]+ | carlinite, jarosite | P3, P31, P32 R3 |
| 147–148 | Rhombohedral rhombohedral tetartohedral |
3 | S6 | 3× | [2+,6+] | dolomite, ilmenite | P3 R3 |
| 149–155 | Trapezohedral | 32 | D3 | 223 | [2,3]+ | abhurite, alpha-quartz (152, 154), cinnabar | P312, P321, P3112, P3121, P3212, P3221 R32 |
| 156–161 | Ditrigonal pyramidal rhombohedral hemimorphic |
3m | C3v | *33 | [3] | schorl, cerite, tourmaline, alunite, lithium tantalate | P3m1, P31m, P3c1, P31c R3m, R3c |
| 162–167 | Hexagonal scalenohedral rhombohedral holohedral |
3m | D3d | 2*3 | [2+,6] | antimony, hematite, corundum, calcite | P31m, P31c, P3m1, P3c1 R3m, R3c |
Hexagonal crystal system
The point groups (crystal classes) in this crystal system are listed below, followed by their representations in Hermann–Mauguin or international notation and Schoenflies notation, and mineral examples, if they exist.[1][9]
| No. | Point group | Example | Space groups | ||||
|---|---|---|---|---|---|---|---|
| Class | Intl | Schoen. | Orb. | Cox. | |||
| 168–173 | Hexagonal pyramidal | 6 | C6 | 66 | [6]+ | nepheline, cancrinite | P6, P61, P65, P62, P64, P63 |
| 174 | Trigonal dipyramidal | 6 | C3h | 3* | [2,3+] | Laurelite and Boric acid | P6 |
| 175–176 | Hexagonal dipyramidal | 6/m | C6h | 6* | [2,6+] | apatite, vanadinite | P6/m, P63/m |
| 177–182 | Hexagonal trapezohedral | 622 | D6 | 226 | [2,6]+ | kalsilite and high quartz | P622, P6122, P6522, P6222, P6422, P6322 |
| 183–186 | Dihexagonal pyramidal | 6mm | C6v | *66 | [6] | greenockite, wurtzite [10] | P6mm, P6cc, P63cm, P63mc |
| 187–190 | Ditrigonal dipyramidal | 6m2 | D3h | *223 | [2,3] | benitoite | P6m2, P6c2, P62m, P62c |
| 191–194 | Dihexagonal dipyramidal | 6/mmm | D6h | *226 | [2,6] | beryl | P6/mmm, P6/mcc, P63/mcm, P63/mmc |
See also
References
- 1 2 Dana, James Dwight; Hurlbut, Cornelius Searle (1959). Dana's Manual of Mineralogy (17th ed.). New York: Chapman Hall. p. 78–89.
- ↑ Ashcroft, Neil W.; Mermin, N. David (1976). Solid State Physics (1st ed.). p. 119. ISBN 0-03-083993-9.
- ↑ Hahn, Theo, ed. (2002). International Tables for Crystallography, Volume A: Space Group Symmetry. A (5th ed.). Berlin, New York: Springer-Verlag. p. 73. doi:10.1107/97809553602060000100. ISBN 978-0-7923-6590-7.
- ↑ http://quantumwise.com/publications/tutorials/item/510-rhombohedral-and-hexagonal-settings-of-trigonal-crystals.
- ↑ http://img.chem.ucl.ac.uk/sgp/medium/sgp.htm
- ↑ Pough, Frederick H.; Peterson, Roger Tory (1998). A Field Guide to Rocks and Minerals. Houghton Mifflin Harcourt. p. 62. ISBN 0-395-91096-X.
- ↑ Hurlbut, Cornelius S.; Klein, Cornelis (1985). Manual of Mineralogy (20th ed.). p. 78–89. ISBN 0-471-80580-7.
- ↑ "Crystallography and Minerals Arranged by Crystal Form". Webmineral.
- ↑ "Crystallography". Webmineral.com. Retrieved 2014-08-03.
- ↑ "Minerals in the Hexagonal crystal system, Dihexagonal Pyramidal class (6mm)". Mindat.org. Retrieved 2014-08-03.