Continuous group action

In topology, a continuous group action on a topological space X is a group action of a topological group G that is continuous: i.e.,

$G \times X \to X, \quad (g, x) \mapsto g \cdot x$

is a continuous map. Together with the group action, X is called a G-space.

If 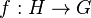$f: H \to G$ is a continuous group homomorphism of topological groups and if X is a G-space, then H can act on X by restriction: 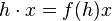$h \cdot x = f(h) x$, making X a H-space. Often f is either an inclusion or a quotient map. In particular, any topological space may be thought of a G-space via 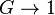$G \to 1$ (and G would act trivially.)

Two basic operations are that of taking the space of points fixed by a subgroup H and that of forming a quotient by H. We write 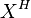$X^H$ for the set of all x in X such that 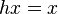$hx = x$. For example, if we write 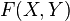$F(X, Y)$ for the set of continuous maps from a G-space X to another G-space Y, then, with the action 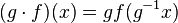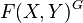$(g \cdot f)(x) = g f(g^{-1} x)$, $F(X, Y)^G$ consists of f such that 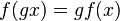$f(g x) = g f(x)$; i.e., f is an equivariant map. We write $F_G(X, Y) = F(X, Y)^G$. Note, for example, for a G-space X and a closed subgroup H, $F_G(G/H, X) = X^H$.

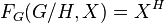References

• John Greenlees, Peter May, Equivariant stable homotopy theory

See also

• Lie group action