Girih

Girih pattern with inlaid floral decoration from Shah-i-Zinda in Semerkand, Uzbekistan

Girih (Persian: گره‎‎, "knot"), also girih sāzī (گره سازی, "knot making") or girih chīnī (گره چینی), is an Islamic decorative art form used in architecture and handicrafts (book covers, tapestry, small metal objects), consisting of geometric lines that form an interlaced strapwork. In Iranian architecture, gereh sazi patterns were seen in banna'i brickwork, stucco, and mosaic faience work.[1] Girih has been defined as "geometric (often star-and-polygon) designs composed upon or generated from arrays of points from which construction lines radiate and at which they intersect."[2]

Straight-edged symmetric shapes are used in girih. Girih typically consists of a strapwork that form 6-, 8-, 10-, or 12-pointed stars separated by polygons and straps, and often they were drawn in an interlacing manner. Such patterns usually consist of a repeating "unit cell" with 2-, 3-, or 6-fold rotational symmetry that tiles the plane with no gaps.[3]

The three-dimensional equivalent of girih is called muqarnas.[4] It is used to decorate the underside of domes or squinches.

History

Further information: Islamic geometric patterns
Mosaic from Roman Theatre (2nd century AD) in Bosra (Syria) displays curvilinear knot patterns that may have been the antecedent of girih patterns.

The girih style of ornamentation is thought to have been inspired by the Syrian Roman knotwork patterns dating back to the 2nd century AD.[1] The predecessors of the girih form were curvilinear interlaced strapwork with three-fold rotational symmetry. The Umayyad Mosque (709–715), in Damascus, Syria has window screens made of interlacing undulating stapwork in the form of six-pointed stars.[5] The window grills in Khirbat al-Mafjar in Jericho (c. 730 AD) has interlaced tracery as well, but with curved lines.[6] Carved stucco showing simple knotted strapwork at the Masjet-e Jami in Nain, Iran (c. 960) also is in the form of curved knotwork.[7] Some of the earliest architectural examples of Islamic geometric patterns made of straight strap lines can be seen in the Arab Ata Mausoleum portal (10th century), village of Tim, near Samarkand, Uzbekistan.[8] and in Ribat-i Mahi, Iran (1019–1020).[9]

Illumination in a Koran (391 AH, 1000–1001 AD) made by Ibn al-Bawwab

Illumination

The earliest form of girih on a book is seen in the fronticepiece of a Koran manuscript from the year 1000, found in Baghdad.[10] This Koran has an illuminated page with interlacing octagons and thuluth calligraphy.[11]

Woodwork

Side of minbar (built in the 13th century) at the Ibn Tulun Mosque.

In woodwork, one of the earliest surviving example of Islamic geometric art is the 13th-century minbar of the Ibn Tulun Mosque in Cairo.[12]

In woodwork, girih patterns can be created by two different methods. In one, a wooden grill with geometric shapes (polygons and stars) would be created first; then the holes would be left either as holes or filled with some material. In the other method, called gereh-chini[13] wooden panels of geometric shapes would be created individually, then combined to create an elaborate design.[1] This woodwork technique was popular during the Safavid period; examples of it can be seen in various historic structures in Esfahan.

Architecture

Girih patterns on walls flanking the portal of the Hunat Hatun Madrasah (Seljuq architecture – circa: 1178–1243) in Kayseri, Turkey.

The term "girih" was used in Turkish as a polygonal strap pattern used in architecture as early as the late 15th century.[14] Also in the late 15th century, girih patterns were compiled by artisans in pattern catalogs such as the Topkapı Scroll.[15]

While curvilinear precedants of girih were seen in the 10th century, fully developed girih patterns are not seen before the 11th century in Iran. It became a dominant design element in the 11th and 12th centuries, as for example, the carved stucco panels with interlaced girih seen in Kharraqan towers (1067) near Qazvin, Iran.[1][16] Stylized plant decoration were sometimes co-ordinated with girih.[17]

After the Safavid period, the use of girih continued in the Seljuk and later the Ilkhanid period. In the 14th century girih became a minor element in the decorative arts and was replaced by vegetal patterns during the Timurid era. However, geometrical strap work patterns continued to be an important element of decorative arts in Central Asian monuments after the Timurid period.[1]

Construction

A girih pattern that can be drawn with compass and straight edge
A window cage at the Topkapı Palace, built with the girih pattern shown at the left.

The first girih patterns were made by copying a pattern template along a regular grid; the pattern was drawn with compass and straightedge. Today, artisans using traditional techniques typically use a pair of dividers to leave an incision mark on a paper sheet that has been left in the sun to become brittle. Straight lines are drawn with a pencil and an unmarked straightedge.[13] Girih patterns made this way are like a two-dimensional crystal, tiling the plane with a unit cell. Because the tiling was done by translation or rotation operations, the unit cells had 2-, 3-, 4- or 6-fold rotational symmetry.[18]

Girih tiles

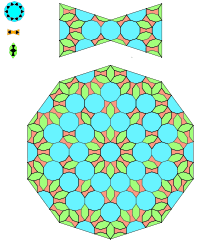
Main article: Girih tiles

From around the year 1200, girih patterns were made with stars and polygon patterns having 5- and 10-fold rotational symmetry. Such figures can be drawn by compass and straightedge as well. However, by the 15th century some girih patterns were not periodic anymore. Such patterns were constructed by the use of girih tiles. These are a set of five tiles with lines drawn on them; when used to tile the plane with no gaps, the lines on the tiles form a girih pattern. It is not yet known when girih tiles were first used instead of compass and straightedge construction. It is considered likely that girih tiles were used on some buildings built around 1200.[19][20]

Two-level designs

Girih pattern on a spandrel from the Darb-i Imam shrine, Isfahan, Iran.
Reconstruction of the larger-scale thick line pattern with larger girih tiles. The outline of the spandrel is shown with yellow dashed line.
The subdivision rule used to generate the girih pattern on the spandrel. The large decagonal and bowtie tiles are subdivided into smaller girih tiles. In the actual spandrel, the decoration lines of the small tiles are filled in black (upper left)

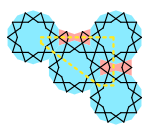
The girih patterns on the Darb-e Imam shrine built in 1453 at Isfahan had a much more complex pattern than previously seen. The details of the pattern indicate that girih tiles, rather than compass and straightedge, were used for decorating the shrine. The patterns on this shrine appear aperiodic; that is, within the area on the wall where they are displayed, they do not form a regularly repeating pattern. Another characteristic of the patterns at the Darb-e Imam shrine is that the girih patterns are drawn at two different scales. There is a pattern that is discernible when the building is viewed from a distance, and a smaller-scale pattern that is part of the larger one, that one sees when near the building.

Although there is evidence that some ancient girih tilings used a subdivision rule to draw a two-level pattern, there are no known historic examples of subdivision rule can be repeated an infinite level of times. For example, the pattern used in spandrel of the Darb-i Imam shrine (see figure) consists of only decagons and bowties, while the subdivision rule uses these two shapes and also the elongated hexagon tile. Therefore, this design does not show self-similarity between level-1 and level-2. However, a tiling design containing docagons and that has self-similarity resulting from the repeated application of a subdivision rule results in a quasi-crystalline tiling (see Aperiodicity section below).

Aperiodicity

A periodic tiling of the plane is the regular repetition of a "unit cell" (the shape, or a group of shapes that is repeated) without any gaps. Such tilings can be seen as a two-dimensional crystal, and because of the crystallographic restriction theorem, the unit cell is restricted to a rotational symmetry of 2-fold, 3-fold, 4-fold, and 6-fold. For this reason, it is not possible to tile the plane periodically with figures having five-fold rotational symmetry, such as a five-pointed star or a decagon. Patterns with infinite perfect quasi-periodic translational order can have crystallographically forbidden rotational symmetries such as pentagonal or decagonal shapes. These shapes with five-fold symmetry repeat periodically, although the space between them contain other shapes that do not have a periodic pattern. Such tilings are called quasi-crystalline tilings.

One way to create quasi-periodic patterns is to create a Penrose tiling. Girih tiles can be subdivided into Penrose tiles called "dart" and "kite", but there is no evidence that this approach was used by medieval artisans.[19] Another way to create quasiperiodic patterns is by subdividing girih tiles repeatedly into smaller girih tiles using a subdivision rule. In the limit the plane would be divided into girih tiles that repeat with frequencies that are aperiodic. The use of such a subdivision rule would have been evidence that Islamic artisans of the 15th century were aware that girih tiles can produce patterns that never exactly repeat themselves. However, all known girih patterns made with girih tiles show no more than a two-level design. In fact, there would have been no practical need to have a girih pattern that had more than two levels of design, because a third level would have a scale either too large or too small to be perceived. It appears that medieval Islamic artisans were using a tool that had the potential of creating highly complex patterns, but they never realized it. As E. Makovicky argues,[21]

The artisans were satisfied by creating a large fundamental domain without being concerned with the mathematical notion of indefinitely expandable quasiperiodic patterns. However, they understood and used to their advantage some of the local geometric properties of the quasi-crystalline patterns they constructed.

The Topkapı Scroll

Panel from the Topkapı Scroll. Black line shows the girih pattern, thin, red lines indicate outline of girih tiles.

The Topkapı Scroll from the late 15th century, documents that girih tiles were used to create girih patterns. The drawings in this pattern book show the girih lines superimposed with the tiles used to generate the girih pattern.[19]

Gallery

References

  1. 1 2 3 4 5 "Gereh Sazi". Encyclopaedia Iranica Online. Retrieved 2013-01-01.
  2. ↑ Terry Allen (2004). "Islamic Art and the Argument from Academic Geometry". Retrieved 2012-01-23.
  3. ↑ Lee, A.J. "Islamic Star Patterns". Muqarnas. 4: 182–197. JSTOR 1523103.
  4. ↑ Rogers, J.M. (1997). "Notes on a recent study of the Topkapı scroll: a review article". Bulletin of the School of Oriental and African Studies, University of London. 60 (3): 433–439. doi:10.1017/s0041977x0003247x. JSTOR 619536.
  5. ↑ "Umayyad Mosque, Damascus, Syria; Window screen, west vestibule". ArchNet. Retrieved 2012-02-12.
  6. ↑ "Khirbat al-Mafjar shiero grill window". Khalili Research Centre. Retrieved 2012-02-12.
  7. ↑ "Friday Mosque of Na'in, Na'in, Iran". ArchNet. Retrieved 2012-02-12.
  8. ↑ "Detail of the Arab Ata Mausoleum portal". ProfiMedia. Retrieved 2012-02-12.
  9. ↑ "Ribat-i Mahi, Ribat-i Mahi, Iran". ArchNet. Retrieved 2012-02-12.
  10. ↑ "Materials and Mediums". Pattern in Islamic Art. Retrieved 2012-02-08.
  11. ↑ The transformation of Islamic art during the Sunni revival. I.B.Tauris. 2002. p. 84. ISBN 978-1-85043-392-7.
  12. ↑ "Jami' Ibn Tulun". Retrieved 2012-02-22.
  13. 1 2 Richard Henry (2007). "Pattern, Cognition and contemplation: Exploring the Geometric Art of Iran" (PDF). Iran Society. Retrieved 2012-02-08.
  14. ↑ Dündar, A. (2003). "Bir Belgeye Göre Amasya II. Bayezid Külliyesi" (PDF). Ankara Üniverstesi İlahiyat Fakültesi Dergisi (in Turkish). 44 (2): 131–172.
  15. ↑ Katz, V.J. (2007). The Mathematics of Egypt, Mesopotamia, China, India, and Islam. Princeton University Press. p. 620. ISBN 978-0-691-11485-9.
  16. ↑ "Architecture – iv. Central Asian". Encyclopaedia Iranica. August 11, 2011. Retrieved 2012-02-08.
  17. ↑ G. A. Pugachenkova, A. H. Dani and Liu Yingsheng (2000). "Urban development and architecture". History of Civilizations of Central Asia Volume IV: The Age of Achievement: A.D. 750 to the End of the Fifteenth Century – Part Two: The Achievements. unesco. ISBN 978-92-3-103654-5.
  18. ↑ Cromwell, P.R. (2009). "The Search for Quasi-Periodicity in Islamic 5-fold Ornament". Mathematical Intelligencer. 31 (1): 36–56. doi:10.1007/s00283-008-9018-6.
  19. 1 2 3 Peter J. Lu and Paul J. Steinhardt (2007). "Decagonal and Quasi-crystalline Tilings in Medieval Islamic Architecture" (PDF). Science. 315 (5815): 1106–1110. Bibcode:2007Sci...315.1106L. doi:10.1126/science.1135491. PMID 17322056.
  20. ↑ Lu and Steinhardt, Supplementary figures
  21. ↑ Emil Makovicky. "Comment on "Decagonal and Quasi-Crystalline Tilings in Medieval Islamic Architecture"". Retrieved 2012-02-22.

Further reading

This article is issued from Wikipedia - version of the 10/11/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.